


The
Online Language Learning Database
OPTICAL
ILLUSIONS
When you think you have an
answer to one of the puzzles below, e-mail it to ![]() and we'll e-mail you back the solution.
and we'll e-mail you back the solution.
DARMO’S PUZZLE
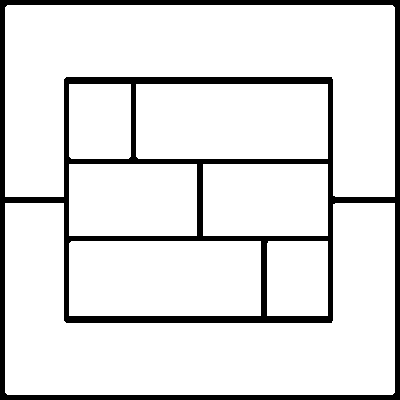
Number the eight areas with 1 through 8 so that consecutive numbers are not adjacent.
LEIBNIZ’S PUZZLE
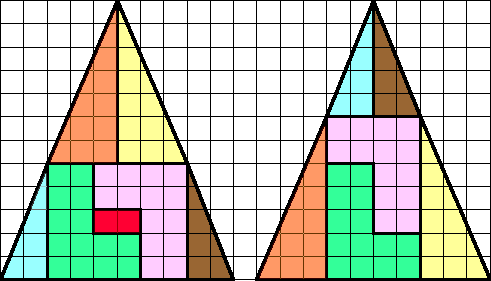
Of the seven pieces on the left,
discard one, and rearrange the remaining
six in the pattern shown on the right. Is there anything wrong
here?
ACE'S PUZZLE

With three straight cuts, divide
a washer into nine pieces.
Leave the pieces in place after
each cut.
(The cuts above form only seven pieces.)
DUNCAN’S PUZZLE

With three straight cuts (they can be at an
angle),
divide
a doughnut into thirteen pieces.
Leave the pieces in place after
each cut.
MY SISTER’S PUZZLE

My sister is three times as old
as I was ten years ago, and she’s twice
as old
as I was when she was my present age. How old is my sister? How old am
I?
Check to see if she's now three times my age ten years ago.
How old was I when she was my age now? Is she twice that?
SHADANIAN’S PUZZLE
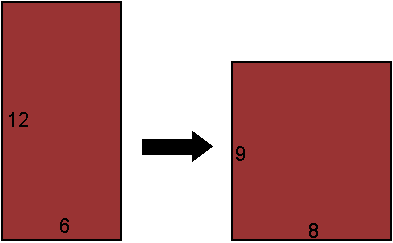
Cut a 6 ´ 12 carpet into two pieces so they can be sewn together into an 8 ´ 9 carpet.
HOLZHAUER’S PUZZLE
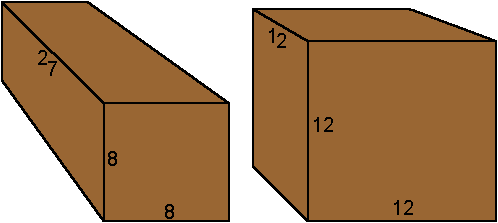
Cut an 8 ´ 8 ´ 27-inch wood beam into
four pieces
so they can be reassembled into a 12-inch cube.
TOM & RAY’S PUZZLE
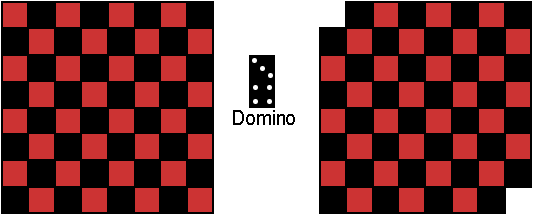
Can you cover the checkerboard
with 32 dominoes? Can you
cover the truncated checkerboard with 31 dominoes?
SHEARSON’S PUZZLE
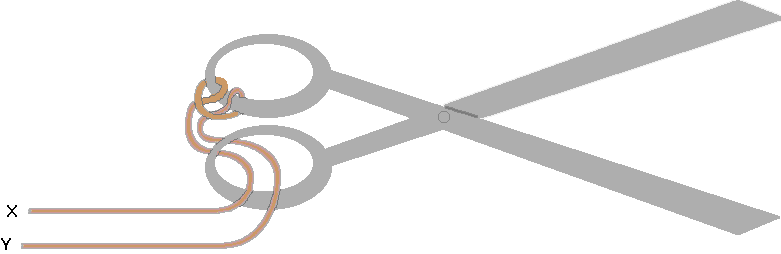
Intertwine a cord with a pair of
scissors as shown. With
ends X and Y anchored, remove the cord from the scissors.
LOUIS’S PUZZLE
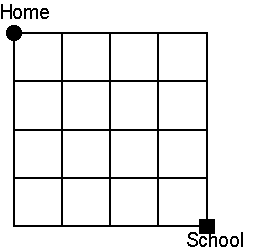
Louis lives four blocks north
and four blocks west of school. How many
different routes can he take to school (always heading south or
east)?
MATT'S PUZZLE
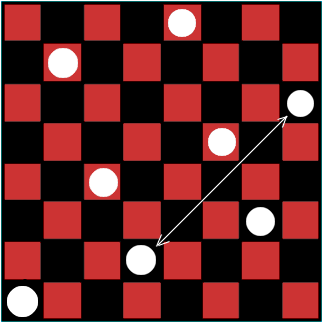
Arrange eight queens on the
chess board so no queen is
attacking another. The arrangement shown above almost
works; two queens still attack each other diagonally.
TERRY’S PUZZLE
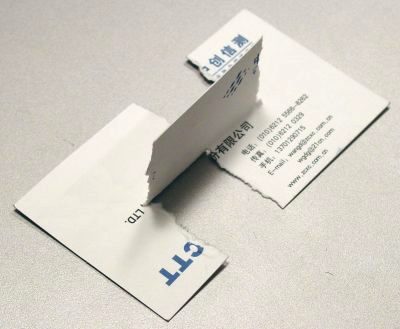
Tear and fold your business card so it looks like this.
RUSS’S PUZZLE
For a sequence of three 1s and 0s, there are eight possible patterns: 000 For a sequence of n 1s and 0s, there are 2n possible patterns. |
For a sequence of three 1s and 0s with no adjacent 1s, there are five possible patterns: 000 For a sequence of n 1s and 0s with no adjacent 1s, how many possible patterns are there? (Try for a few values of n, see a pattern, and generalize. Then prove.) |
ARNIE’S PUZZLE

Arnie has opened a 12-oz can of
soda in the car. (The can itself weighs 1/2 oz.)
If he drinks nothing, the center of gravity is at the center of
the can. If he drinks
it all, the center of gravity is again at the center of the can.
How much soda should
Arnie drink so the can has the least chance of tipping over if he
comes to a sudden
stop? (Assume a fluid ounce weighs an ounce.)
HOWARD’S PUZZLE

A stick is broken at random in
two places. What
are the odds that you can form a triangle of the pieces?
LIBRO’S PUZZLE
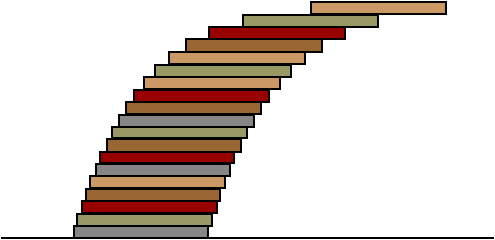
What is the minimum number of
books in a skewed stack so the
top book projects one book width beyond the bottom book?
How many so it projects two book widths?
BINNY'S PUZZLE

Pick four weights so some
combination of them in the left pan
will balance a sack of wheat from 1 to 15 lb. by 1-lb.
increments.
TRYON'S PUZZLE
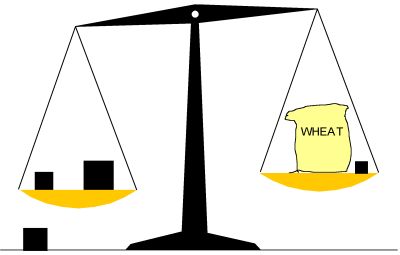
Pick four weights so some
combination of them in the left and right
pans will balance a sack of wheat from 1 to 40 lb. by 1-lb.
increments.
JIMMY'S PUZZLE
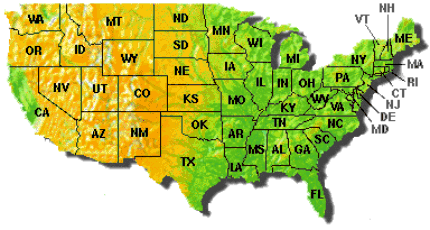
| Jimmy, in a west-coast state, calls
his mother in an east-coast state. After a while she says she really should be getting to bed, and mentions the time. "Hey!" says Jimmy, "it's the same time here!" What states are they in? (There is no trick in the wording here. A "west-coast state" is Washington, Oregon, or California, and an "east-coast state" is one from Maine to Florida. "The same time" means what the clock reads in that time zone when correctly set to standard or daylight-saving time, according to the time of year.) |
GRENZA'S PUZZLE
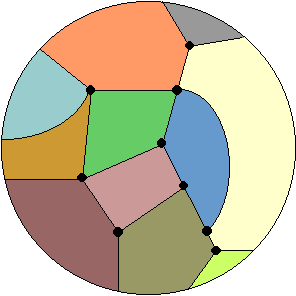
A planet with no bodies of water is divided into countries by lines that meet (terminate) at points. No country is an "island" (surrounded by one country). There are 37 lines and 15 points. How many countries are there?
PIERRE'S PUZZLE
Pierre has thrown several pieces of 2×4 lumber into the lake. Some pieces float with the 4" side up and some with the 2" side up. What is the deciding factor?
RACHEL'S PUZZLE
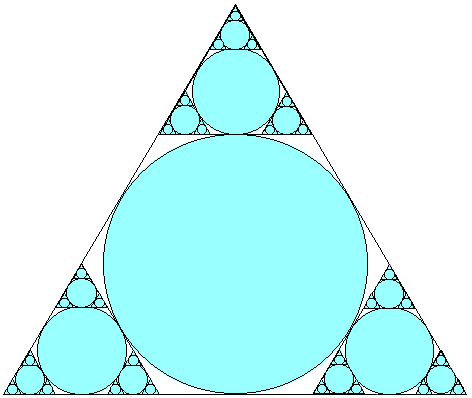
There is an infinite number of
circles. What fraction
of the area within the triangle do the circles occupy?
DAN’S PUZZLE
x y = y x
For a given real x greater
than one, the equation almost |
GREG'S PUZZLE
Bisect a line segment using only a compass (no straight edge).
MARTIN'S PUZZLE
| Disprove the assertion that |
CUBBY'S PUZZLE
| Prove the assertion that |
PETTIFERM'S PUZZLE
It seems that 2p–1 – 1 is
always divisible by p if p is prime. |